Golden Ratio - The Secret To Aesthetics?
This post is for the graphic designers, photographers, architects, and other "form" designers reading my blog.If you haven't heard of the Golden Ratio (Also known as: Phi, Golden Section, Golden Number, Golden Cut, Golden Proportion, Golden Mean, Extreme and Mean Ratio, Mean of Phidias, Medial Section, Divine Section, and Divine Proportion. Whew!) then you should really take a moment to review this post, as I will be sharing information and resources pertaining to the Golden Ratio.
For simplicity, I will refer to it only as Golden Ratio in this post.
Since discovering & researching the Golden Ratio, I can sincerely say that my aesthetics has improved dramatically! Not to mention (by utilizing some of the tools I'm about to share with you) the improved productivity I have experienced!
So what is the Golden Ratio?
Golden Ratio is an irrational mathematical constant that represents the ratio of one quantity to another quantity. Golden Ratio is given the value of 1.6180339887... and continues on infinitely. You can find this value in nature, universal bodies (such as galaxies), artwork, architecture, literature, and many other areas of interest. To some it is merely an insignificant coincidence, to others it is a number of vast importance - interconnecting life, matter, and the universe.
 In this illustration, you see a large rectangle divided by it's Golden Ratio. The smaller of these areas is then
In this illustration, you see a large rectangle divided by it's Golden Ratio. The smaller of these areas is thendivided by it's own Golden Ratio. This continues until you get the resulting divisions of the original rectangle.
Brief History of the Golden Ratio
You can find the Golden Ratio in all corners of ancient architecture, literature, and artwork. However, discovery of the Golden Ratio is subjectively attributed to Pythagoras, the creator of Pythagorean Theorem. If you research into Pythagorean Theorem, you'll run across references to Euclid, the Father of Geometry, which also verified the theorem in his writing Euclid Elements. Coincidentally, this writing, Euclid Elements, provides the first definition of the Golden Ratio.
Below is a great example of the relationship between the Golden Ratio and the Pythagorean Theorem. I stumbled across this while researching and thought it was interesting.
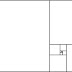
 The purple vertical that passes through point A represents the Golden Ratio of the bottom box. The top boxes represent the same area as the same colored areas of the bottom box. The Golden Ratio of this bottom box forms congruency with all areas as well as the Pythagorean triangle.
The purple vertical that passes through point A represents the Golden Ratio of the bottom box. The top boxes represent the same area as the same colored areas of the bottom box. The Golden Ratio of this bottom box forms congruency with all areas as well as the Pythagorean triangle. Golden Ratio, although not widely known by the general public like Pythagorean Theorem, has been used in structural design and art by architects, philosophers, and artists for thousands of years.
The most famous of these would probably be Leonardo Da Vinci. Looking into his artworks, you will find numerous correlations between his work and the Golden Ratio. I will display demonstrations of this later in this post which will present multiple artworks that apply Golden Ratio. First, I'd like to present why this number is so significant to all these great thinkers and philosophers of our past history.
Golden Ratio in Nature
You can find the Golden Ratio in many aspects of nature. Some say you can even find it in our own anatomical make-up. The distance from finger tip to the wrist in relation to the distance from the wrist to the elbow is very close to Golden Ratio. You can find similar representations of Golden Ratio throughout human anatomy.
Now, to fully understand the Golden Ratio in nature, you'll also need to have a little knowledge of the Fibonacci Spiral. I don't want to get too off topic here, but the Fibonacci Spiral is interrelated with the Golden Ratio very deeply. Below shows you how the Fibonacci Spiral is created using the Golden Ratio.
 Building on the previous illustration of a rectangle divided by it's Golden Ratios, we have connected adjacent
Building on the previous illustration of a rectangle divided by it's Golden Ratios, we have connected adjacentcorners of each rectangle with a one-quarter circle radius. This forms what is known as the Fibonacci Spiral.
 A Nautilus Shell has similar properties to the Fibonacci Spiral
A Nautilus Shell has similar properties to the Fibonacci Spiral A cacti with multiple Fibonacci-like Spirals.
A cacti with multiple Fibonacci-like Spirals.The natural growth of this plant is similar to a Fibonnaci Spiral.
Most of these examples are "close" to Golden Ratio, however none of them are precisely .6180339... There are also plenty of examples in nature that don't portray any relation to Golden Ratio. It is a matter of belief on how you choose to look at Golden Ratio. I however, do find it significant that throughout nature you can find a ratio that is VERY CLOSE to Golden Ratio.
Golden Ratio in Art
As you research more into Golden Ratio, you will conclude that this number was common knowledge all throughout our history. The Greeks, Romans, Egyptians, Chinese, and nearly every other civilization portray Golden Ratio in their artwork. Leonardo Da Vinci, who revolutionized art theory during the Renaissance, perfected it's use in every single painting he did. One would conclude that he was in fact obsessive about applying the Golden Ratio to his work.
 Leonardo Da Vinci's Vitruvian Man displays the use of Golden Ratio in the human body.
Leonardo Da Vinci's Vitruvian Man displays the use of Golden Ratio in the human body. Leonardo Da Vinci's Mona Lisa is probably the most well known and popular painting in
Leonardo Da Vinci's Mona Lisa is probably the most well known and popular painting inhuman history. It's no wonder - every single aspect of this painting was done in Golden Ratio.
 The Great Wave Off Kanagawa by Japanese Artist Hokusai was drawn with ratios equal to the Fibonacci Spiral and Golden Ratio.
The Great Wave Off Kanagawa by Japanese Artist Hokusai was drawn with ratios equal to the Fibonacci Spiral and Golden Ratio. Ancient Egyptian Architect Khesi-Ra’s panel was carved to pass on his theories of
Ancient Egyptian Architect Khesi-Ra’s panel was carved to pass on his theories ofharmonious geometry. It elegantly displays numerous representations of Golden Ratio.
Golden Ratio in Architecture
Architecture is at the heart of human innovation. From the pyramids in Egypt to the Parthenon in Greece, there are many examples of the Golden Ratio.
Conclusion
While some may dispute the significance of the Golden Ratio, it is apparent that through our history there has been a fascination with it. Many will speculate on the validity of it in nature, as well as in our history. It's important to realize that, while although some of these examples are in fact not quite perfect to Golden Ratio, there still is a significance to the approximate value. I have come to conclude that it is not the "holy grail" of numbers, but merely a great measurement to start from. There happens to be an uncanny appeal to the aesthetics of this ratio and I intend to keep using it in my works. If you are looking for an improvement in your own aesthetics, Golden Ratio will be a great resource.
Golden Ratio Resources
Here I have gathered a few resources that you can use to implement and observe Golden Ratio practices. If you have any others you would like to share, I will add them here. Enjoy!
Atrise Golden Section - http://www.atrise.com/golden-section
I love this tool as it allows me to utilize many different views. What this tool does is overlays a Golden Ratio grid (as well as a few other views) on top of all your windows and can be scalable to your liking. I particularly use the Ratio section view for any spacing I am doing with typography and/or geometrical shapes. This saves me a lot of time perfecting the spacing of elements in my design.
Phiculator - http://www.thismanslife.co.uk/phiculator
A great little calculator for quickly getting a number's Golden Ratio value.
Sources of Information
Golden Ratio in Wikipedia
Pi, Phi, and the Great Pyramids
Golden Ratio in Geometry
Fibonacci Numbers, The Golden Section, & The Golden String










14 comments: